Alternator done, now chosing the air gap size

[elt]

I've come up with these numbers and "conclusions" based on the math I've gleamed from what I've read... but this is my first mill and I have zero experience with what the numbers really mean. Any help from folks that know what's going on (unlike me!) would greatly appreciated. Thank you, - Ed.

I'd like to get 250 watts in a 12.5 mph wind. Betz limit at 12.5 mph is about 5.8 watts per square foot. It's suggested that a "good" turbine will get about 60% of that; I hope to build a good turbine! 250 watts / 5.8 / .6 /  pi and take the square root of that for the radius of the needed blade equals 4.8 feet radius... so that's where I got the idea of a 10 foot mill.

Advice here was to build the alternator first, though, before carving blades so I did. Here's a picture of my alternator -

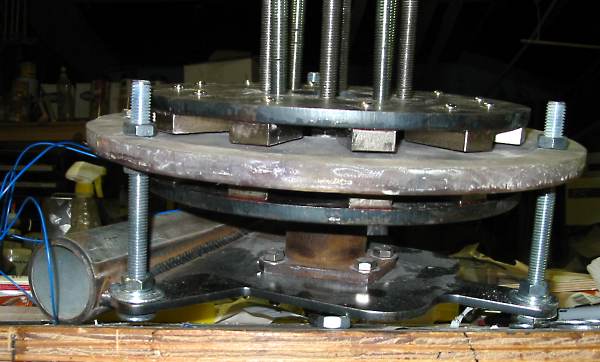
It has 24 1x2x.5 N42 neo's on 3/8" thick steel rotors.

The nine stator windings are #15, two in hand, 48 turns and 1/2 inch thick. The casting is 9/16" thick. The winding resistance is .3 ohms (based on 35' of wire). I originally did my test coil with a 7/8" air gap but the stator came out nice and flat so I did "final" testing with both a 3/4" air gap and a 7/8" air gap.

(Note: there's fewer turns than traditional in the coils for a 24 volt system because I want a better match and less heat at higher wind speeds; I'll use a boost converter to get charging current out of the coils at lower wind speeds.)

Testing the alternator with a 3-phase rectifier with 300 ohm resistor on the output, I calculate 25 volts out at

 210 rpm with a 3/4" air gap, and

 245 rpm with a 7/8" air gap.

Assuming a 10' blade with a TSR of 7, cut-in mph for 25 volts are

 10.5 mph with a 3/4" air gap, and

 12.3 mph with a 7/8" air gap.

How much power?

Using the method for calculation power described by flux, the mill with a 3/4" gap would produce

  855w @ 17 mph, and

 1262w @ 20 mph

Total output from the alternator would be 1262w to the battery plus 1180w in heat in the stator for a total of 2442 watts. Besides being concerned that the stator would melt in 20+ winds, I don't think that the mill would get there... power out for a "good" turbine (.6 x Benz limit) at 20 mph is 14 watts/sq-ft. 2442/14 = 175 sq-ft which would be a 15 foot mill!

The mill with a 7/8" gap would produce

530. watts at 17 mph
     
866. watts at 20 mph
     
     

Total output from the alternator would be 866w to the battery plus 564w in heat in the stator for a total of 1430 watts. 1430/14 = 102 sq-ft which would be a 11.5 foot mill...

On the other hand, the 530 watts + 220 watts of heat at 17 mph = 750 watts divided by 8.59 watts per sq-ft for a "good" turbine in 17 mph wind yields a requirement for a 10.5" diameter blade... seems like it would just be starting to stall.

--%--

I'd like the mill to furl somewhere shortly after 20 mph winds.

I don't know whether my notion of stalling based on "how big a blade is needed for the power output" is really how stall is estimated or whether I should be stalling a little bit (but not a lot) or not before furling.

My gut feel is that the gap should be at least 7/8" but I don't know if I should make it a little bit bigger...

Thanks a lot!

- Ed.

[elt]

... or maybe a lower TSR would slow down the alternator to match output at a given wind speed?

- Ed.

[Flux]

Boy this is getting serious stuff, you will be checking with an anemometer soon , then we shall be in trouble.

 No honestly I think you will struggle to manage 60% of Betz, that is about as much as I would expect from the prop at 12 mph, doesn't leave much for the alternator and converter. In reality you shouldn't be too far off that but that figure is about on the practical limit.

You are certainly in the right region, my 10ft machine cuts in direct at 220 rpm.

If your line resistance is negligible then you would probably be best with the 7/8 gap but to be honest you will not see significant stall with the 3/4 gap. The prop will adapt quite well until you drag it below tsr5. If you are like me you may appreciate the quieter running more than the extra 50W at 20 mph.

I am not sure how you arrived at the losses, I think you will still be up over 60% efficiency at 1kW out so the losses will be well down on what your are predicting ( probably under 600W).

If you have negligible line resistance use the 7/8 gap, otherwise try it at 3/4.

I think you will do well.

flux

[elt]

I am not sure how you arrived at the losses, [...]

Well, I think that I get different numbers every time I do the math... this time's no different! Ad I also realize that my numbers are based on linear rpm/windspeed relationship and don't take into account that the TSR of the dlade will start going down at higher windspeed when the alternator loads up... but here goes -

With a 25 volt cut-in at 245 rpm, 12.3 mph at TSR 7 for the 7/8" air gap, the turbine is making 25.8 volts. (.8v for the diode drop.) Assuming that the prop kept up, the voltage at 20 mph would be 25.8 * 20/12.3 = 42 volts.

26 volts go into the diode and battery (diode drop is 1v with more current going through it) so 42-26= 16 volts dissipated in the coils. The leg resistance is .3 ohm, that times your '1.3 fudge factor" is .4 ohms so amps = 16/.4 = 40 amps.

At 40 amps, the heat in the coil is 40x40x.4 = 640 watts.

At 40 amps, the heat in diodes is (2/3x40) x 3 = 80 watts

at 40 amps and 24 volts, 960 amps go to the battery.

= 1680 watts. (feed line losses would subtract from the coil losses, total watts would still be the same.) So, yes, efficiency will be about 60%.

But it would take a 1680/14 = 120 sq-ft prop, 12.4 foot blade to get that much power in a 20 mph wind. A 10 foot blade only has 64% of that area so its not going to be able to run that fast.

So 1680 (total) watts in 20 mph wind isn't going to happen... what does/will happen?

From here on I'm pretty much guessing but I'm going to try to figure it out based on power, RPMs and TSRs...

So the 10 foot blade will only make .64 x 1720 =  1100 watts in a 20 mph wind.

Since watts go as the cube of the wind speed, 65.4% of the power would come from (an equivalent of) the cube_root(.64) = 86.8% of the TSR 7, 20 mph RPMs so the blades would be working at a TSR of .86 x 7 = TSR 6 ?

Since rpm would 86%  of the original estimate at 20 mph, the voltage would also be reduced by that amount .86 x 42 = 36.5 volts.

Doing the math again -

26. volts go into the battery so 10.5 volts goes into stator heat.
    
10. 5 volts /.4 ohms = 26 amps
    
    

coil loss is 274 watts

diode loss 52 watts

battery charge is 654 watts.

= 960 watts ... not exactly the 1100 watts the first guess gave me but now the efficiency is quite a bit more than the previous 60% so I guess that it'll still make the 1100 watts (total) and not slow down quite as much as my first guess.

Does that sound more or less on track?

Thanks again,

- Ed.

[Flux]

OK I can see where you are coming from. Firstly without mppt the prop will not track wind speed ( tsr will fall if you prefer to look at it that way)

The second thing is that the 1.3 factor is only useful for the effective resistance to get a figure for current out. Don't use it for power. Even using dc current and alternator resistance without the 1.3 factor gives high results but is near.

A better way to get at power loss is to use rms current squared a resistance of one phase ( half line resistance ) to get phase power and multiply by three. The rms current will be roughly 10/13 x dc current.

Don't get too serious with any of these calculations, there are too many factors, at best you can only get an approximation.

The prediction of current from speed and winding resistance is quite good if you include the 1.3 factor. Power loss is fairly good if you use the 3 times power per phase method, but any predictions from prop power is really stretching things beyond reasonable realms of certainty. I don't think anyone has done any measurements sufficiently comprehensive and accurate to really know what goes on there and it would be blade specific. your blades may not behave as mine or Dan's.

The shape of the power curve with tsr is very much in the land of guesswork. My experiments with MPPT and power curve measurements indicate that my blades want to run at lower tsr in high winds, but that may not be true of other blades.

All I can say is that you are in the right region and it should work well. You will see more power in wind speed above about 12 mph compared with the conventional approach and you will have less stator heat. You can't hope for perfect matching but it will be far better than the normal bogged down into stall method.

Flux

[elt]

Don't get too serious with any of these calculations, there are too many factors, [...] You will see more power in wind speed above about 12 mph compared with the conventional approach and you will have less stator heat.

 ... you're right, I don't believe that I'd get exact numbers; whatever they are, I can see that they will be better than the conventional approach (for my site with higher winds.)

Thanks again for encouraging me down this path.

- Ed.